Оценки первого собственного значения эллиптической краевой задачи с параметром
11
2
2
0
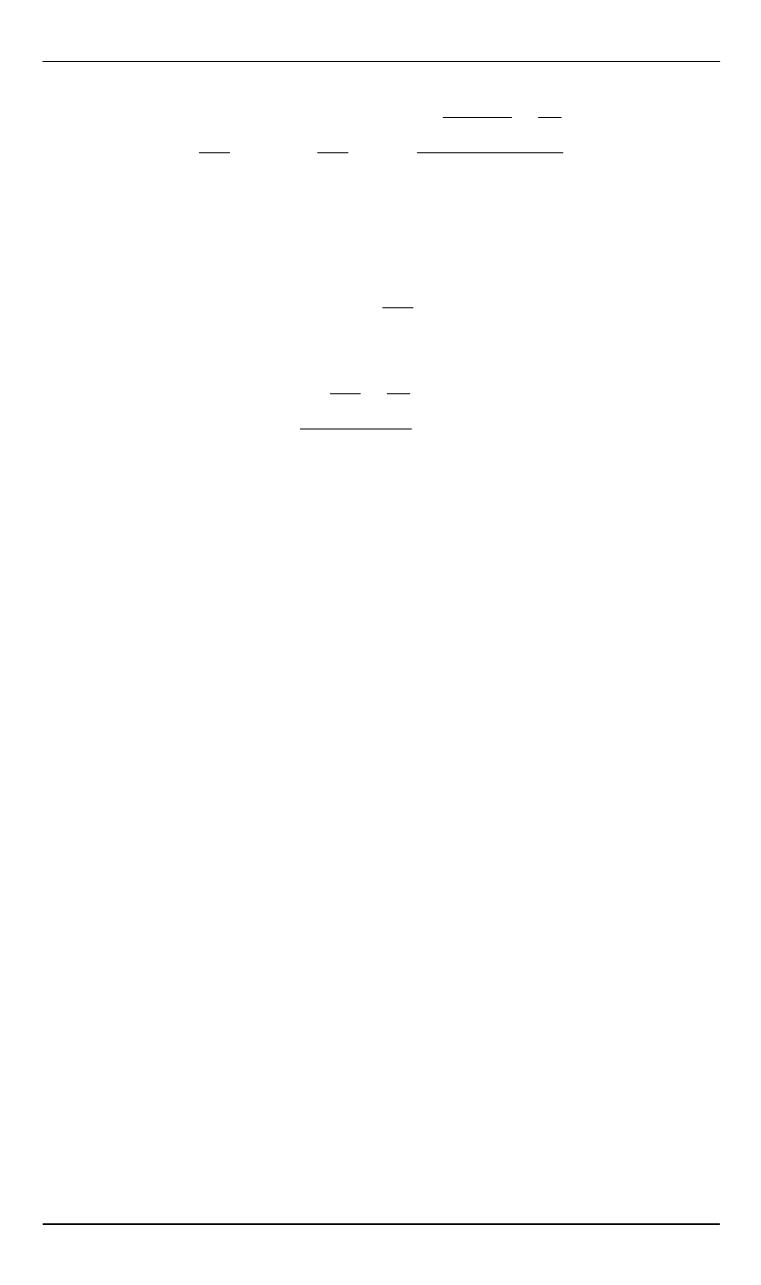
( , 0)
(0) lim ( )
( , 0)
k
z x
ds
N g
d
d k
dk
dk
z x dx
и функция
( )
k
имеет асимптотическое разложение
( )
(0)
(0) ( )
d
k
k
o k
dk
2
( )
1
2
( ),
0.
d
u ds
N g
k
o k k
u dx
(26)
Разложение (26) доказывает соотношение (20).
Исследование выполнено при финансовой поддержке гранта
РФФИ № 11-01-00989.
ЛИТЕРАТУРА
1. Ladyzhenskaya O. A.
The Boundary Value Problems of Mathematical Physics
.
Berlin, Springer-Verlag, 1985, 356 p.
2. Courant R., Hilbert D
. Methods of Mathematical Physics
. New York, Wile, 1989,
vol. 1, 560 p.
3. Kato T.
Perturbation theory for linear operators
. Berlin, Springer-Verlag, 1995,
642 p.
4. Кондратьев В.А., Ландис Е.М. Качественная теория линейных дифферен-
циальных уравнений в частных производных второго порядка.
Итоги науки
и техники. Современные проблемы математики. Фундаментальные
направления
. Москва, ВИНИТИ, т. 32, 1988, с. 99– 218.
5. Sperb R. Untere und Obere Schranken fur den Tiefsten Eigenwert Elastisch
Gestutzen Membran.
Zeitschrift Angew
.
Math. Phys
, 1972, vol. 23, no. 2,
ss. 231–244.
6. Daners D., Kennedy J.B. On the Asymptotic Behaviour of the Eigenvalues
of a Robin Problem.
Differential Integral Equations
, 2010, vol. 23, no. 7–8.
pp. 659–669.
7. Hintermuller M., Kao C.-Y., Laurain A. Principal Eigenvalue Minimization for
an Elliptic Problem with Indefinite Weight and Robin Boundary Conditions.
Appl. Math. Optim
, 2012, vol. 65, no. 1, pp. 111–146.
8. Giorgi T., Smits R. Monotonicity Results for the Principal Eigenvalue of
the Generalized Robin Problem.
Illinois J. Math
, 2005, vol. 49, no. 4,
pp. 1133–1143.
9. Giorgi T., Smits R. Bounds and Monotonicity for the Generalized Robin
Problem.
Z. Angew. Math. Phys
, 2008, vol. 59, no. 4, pp. 600–618.


